Squares Sudoku
In addition to the normal Sudoku rules, there is one additional rule for a Squares Sudoku puzzle - sum of the numbers in each cage should be a perfect square.
Squares Sudoku puzzle
Here is a hard Squares Sudoku puzzle 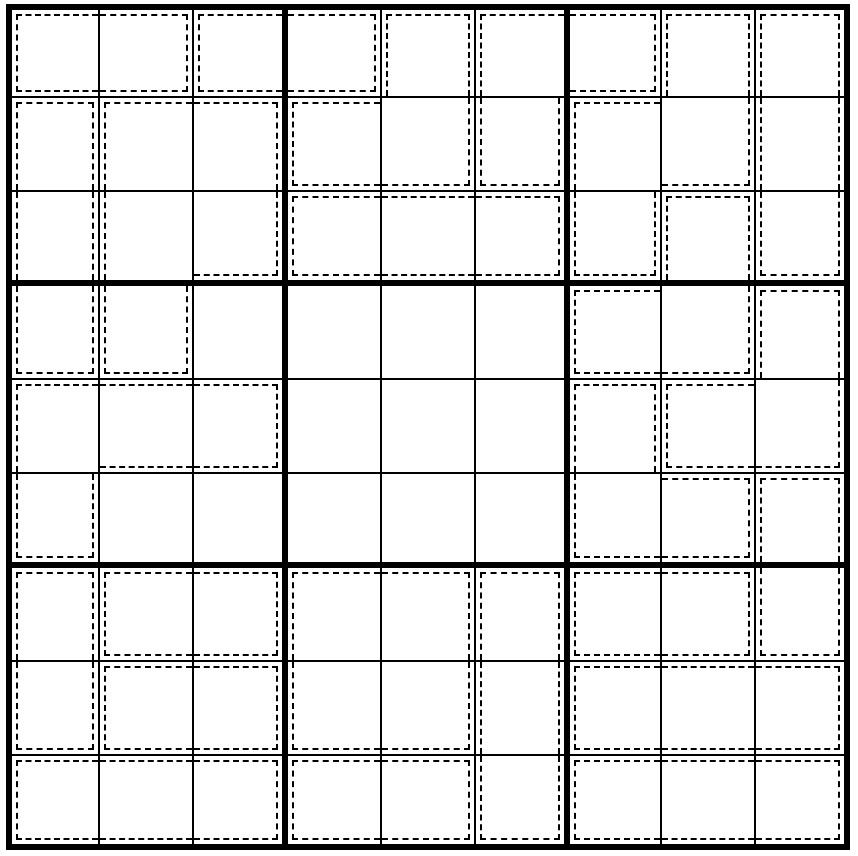
Solution using Z3
from z3 import Solver, And, Int, Distinct, sat, If, Or
puzzle = [
[(0,0),(0,1)],
[(0,2),(0,3)],
[(0,4),(1,3),(1,4)],
[(0,5),(1,5),(0,6)],
[(0,7),(1,7),(1,6),(2,6)],
[(0,8),(1,8),(2,8)],
[(1,0),(2,0),(3,0)],
[(1,1),(2,1),(3,1),(1,2),(2,2)],
[(2,3),(2,4),(2,5)],
[(3,6),(3,7),(2,7)],
[(4,0),(4,1),(4,2),(5,0)],
[(4,6),(5,6),(5,7)],
[(4,7),(4,8),(3,8)],
[(5,8),(6,8)],
[(6,0),(7,0)],
[(6,1),(6,2)],
[(6,3),(6,4),(7,3),(7,4)],
[(6,5),(7,5),(8,5)],
[(6,6),(6,7)],
[(7,1),(7,2)],
[(7,6),(7,7),(7,8)],
[(8,0),(8,1),(8,2)],
[(8,3),(8,4)],
[(8,6),(8,7),(8,8)],
]
def print_grid(mod, x, rows, cols):
for i in range(rows):
print(" ".join([str(mod.eval(x[i][j])) for j in range(cols)]))
def solveSqudoku(puzzle, n):
X = [[Int("x_%s_%s" % (i+1, j+1)) for j in range(n)] for i in range(n)]
# each cell contains a value in {1, ..., n}
cells_c = [And(1 <= X[i][j], X[i][j] <= n) for i in range(n)
for j in range(n)]
# each row contains a digit at most once
rows_c = [Distinct(X[i]) for i in range(n)]
# each column contains a digit at most once
cols_c = [Distinct([X[i][j] for i in range(n)]) for j in range(n)]
# each 3x3 square contains a digit at most once
sq_c = [ Distinct([ X[3*i0 + i][3*j0 + j]
for i in range(3) for j in range(3) ])
for i0 in range(3) for j0 in range(3) ]
# sum of numbers in each cage is a square
puzz_c =[]
for cage in puzzle:
cs = sum([X[i][j] for i,j in cage])
puzz_c.append(Or([(cs==k) for k in [4, 9, 16, 25]]))
squdoku_c = cells_c + rows_c + cols_c + [And(puzz_c)] + sq_c
s = Solver()
s.add(squdoku_c)
if s.check() == sat:
m = s.model()
print("Here is the solution")
print_grid(m, X, n, n)
else:
print("Failed to solve the puzzle")
solveSqudoku(puzzle, 9)Here is the solution:
6 3 4 5 9 1 8 7 2
8 2 1 3 4 5 7 9 6
5 7 9 8 2 6 4 3 1
3 6 7 2 1 8 9 4 5
1 9 2 7 5 4 6 8 3
4 5 8 9 6 3 1 2 7
7 4 5 6 8 2 3 1 9
9 1 3 4 7 5 2 6 8
2 8 6 1 3 9 7 5 4