Hamiltonian Cycles

Matchstick graph

While investigating whether a $54$ vertex quartic graph has a Hamiltonian cycle in the context of a recreational math problem, I came across this theorem of Tutte, which says that every $4$-connected planar graph has a Hamiltonian cycle. I was unsure if a $54$ vertex quartic graph could be planar, so I googled “graph 54 vertices 108 edges”, lo and behold, here is the beautiful matchstick graph and the Hamiltonian cycle that I found 😊.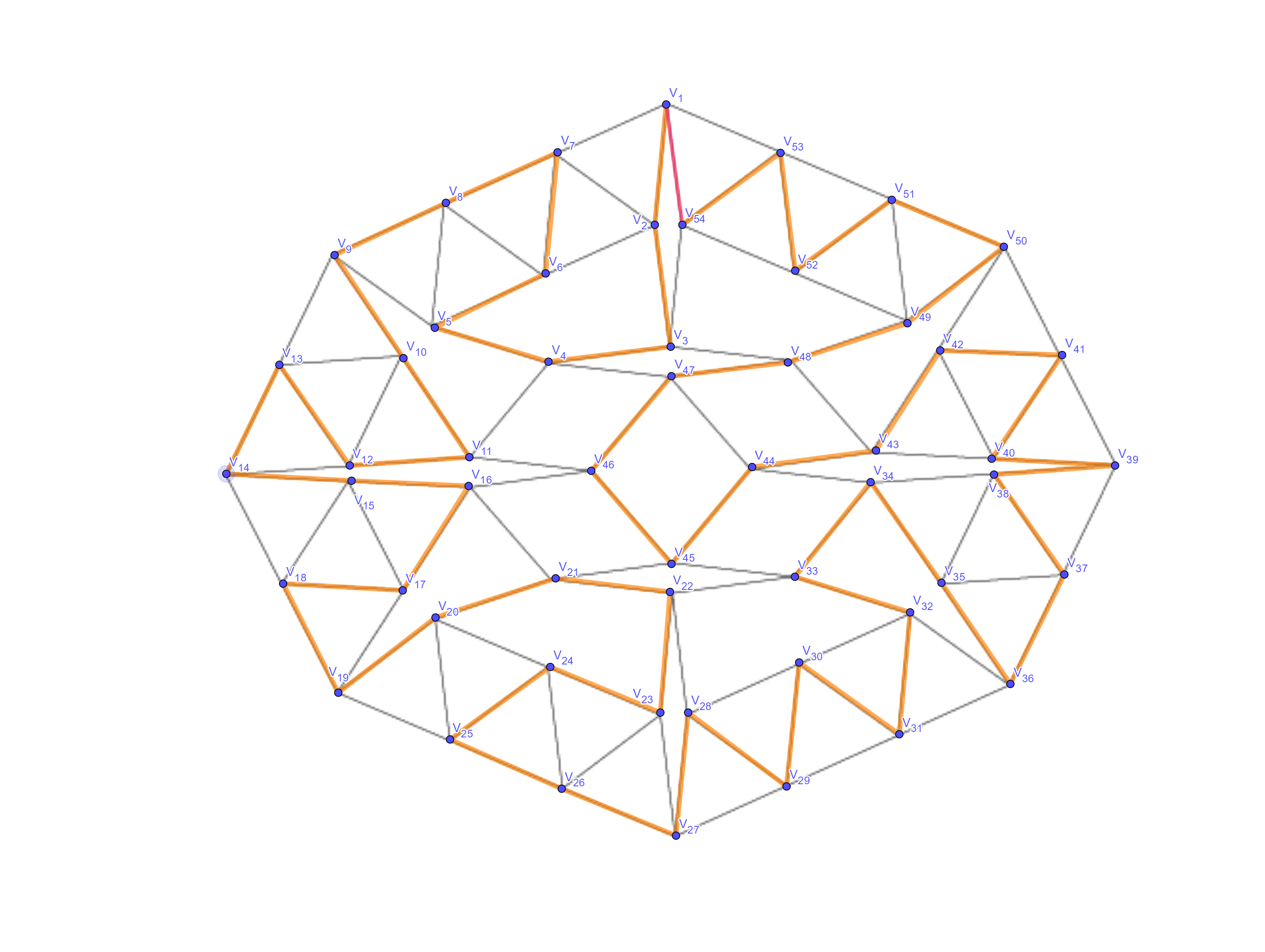

Problem

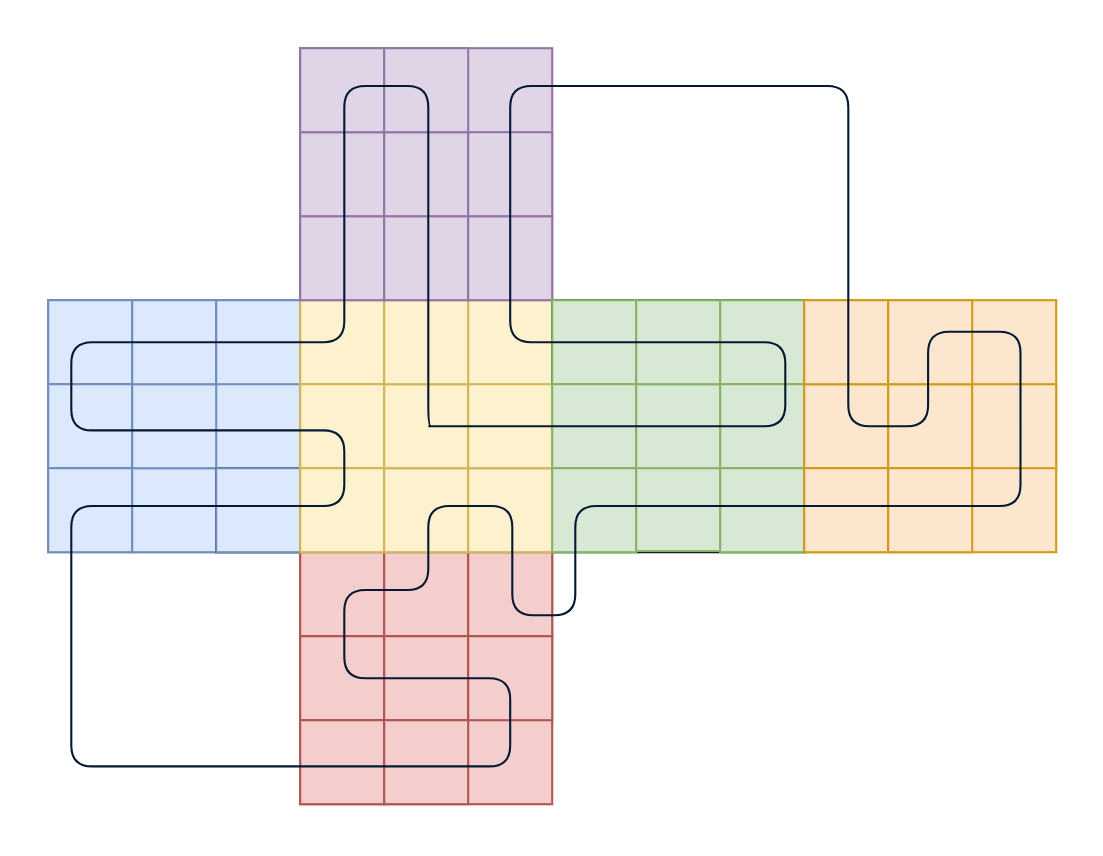
On the surface of a $3\times3$ cube is it possible to walk a path starting in one surface square, walking to neighbouring squares, visiting each of the $54$ squares only once? Is there a circuit?

Solution

Proof without words 😊